The remarkableness of the scale tones can be accounted for in terms of melodic charge of the scale tones. In this figure the tones are arranged around the circle of fifths, transposed such that all fall in the same octave. Listen to them by pointing at them. For each tone you hear first the scale of the key and then the tone.
Now listen to how the melodic charge is marked by the performance rules. The duration, loudness and vibrato extent of the tones vary according to the melodic charge.
Here is our example, the theme of the first Kyrie in J S Bach's b-minor Mass.
Compare No-Melodic-Charge, Exaggerated and Medium application of the rule. Also try the Inverted version, where the marking is greatest for the trivial tones.
In this example another rule is applied as well: Phrasing marking phrases and subphrases.
Example: J S Bach, Fugue theme of the Kyrie movement from the b-minor Mass, BWV 232.
| k = 0 | |||
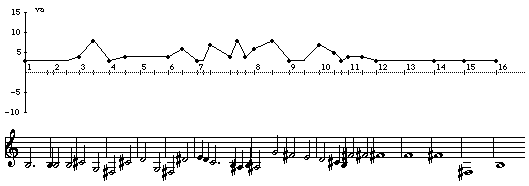
| k = 1.5 | |||
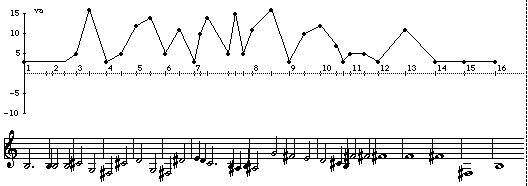
| k = 3.0 | |||
| k = -3.0 |
The graph above the melody shows the vibrato extent of the different tones, which is proportional to their melodic charge values.
| No-Melodic-Charge, k = 0 | |
|---|---|
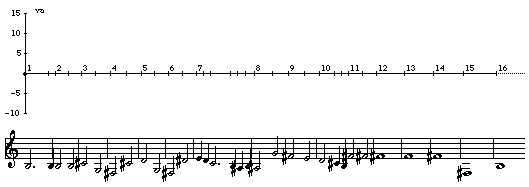 |
|
| Medium, k = 1.5 | |
|---|---|
 |
|
| Exaggerated, k = 3.0 | |
|---|---|
 |
|
| Inverted, k = -3.0 | |
|---|---|
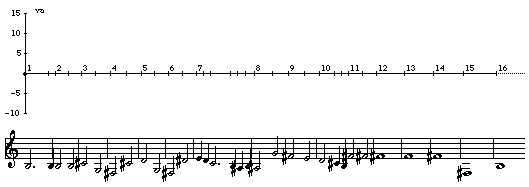 |
|