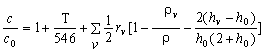PREDICTION AND MEASUREMENTS OF EXHALED AIR EFFECTS

IN THE PITCH OF WIND INSTRUMENTS

Leonardo Fuks (1,2)

 (1) Dept. of Speech, Music and Hearing, Royal Institute of Technology, S-10044, Stockholm, SWEDEN

leonardo@speech.kth.se

(2) Escola de Música, Universidade Federal do Rio de Janeiro, R. do Passeio 98, 200021-290 BRAZIL

Published in Proceedings of the Institute of Acoustics, Vol 19 Part 5, Book 2:

373-378 (1997), St Albans, United Kingdom

1. Introduction

The natural resonance frequencies of wind instruments are dependent on the geometry of the air column, the dimensions and design of reed/mouthpiece, the placement and size of toneholes and the sound speed in the gas inside the instrument. Also, this gas continuously changes in composition with respect to the content of carbon dioxide (CO₂) and oxygen gas (O₂) due to the player’s metabolic activity. These changes should affect the fundamental frequency to some extent. This frequency is also a function of the embouchure configuration and the highly varying blowing pressures (Porter, [1]; Bak and Doemler [2], Fuks & Sundberg, [3]). The purpose of the present study was to provide an experimental and theoretical basis for analysis and measurement of the impact of the gas changes in the sounding pitch.

Typical atmospheric air contains a concentration of CO₂ ranging between 0.03% and 0.06%, while O₂ is found at a percentage of approximately 20.9 %, regardless of local altitude (e.g., Zenz, [4]).These are the gases whose percentages change between inhaled and exhaled air. In addition, there is metabolic production of water, which is expelled as saturated vapour. It is also important to note that the respiratory airways comprise a volume defined between the air inlet and the first pulmonary structures, called anatomical dead space, in which the air practically does not change in composition from the ambient values. The anatomical dead space is in average ca. 150 ml. Variations in exhaled CO₂ during performance of a solo work with the oboe and sustained tones with the clarinet have been documented by Fuks [5]. The results showed that in wind instrument playing, the CO₂ contents in the pulmonary air may vary considerably with time, roughly from 2.5% just after a deep breath up to 8.5% after a long playing period of ca one minute. The O₂ contents in the alveolar gas, thus the air to be exhaled, are reported to achieve a minimum value of 14% (Schmidt & Thews, [6]).

2. SOUND SPEED AS A FUNCTION OF GAS CHANGES

The effect of temperature on the sound speed for exhaled air has been described in detail by Nederveen [7], who assumed a saturated air mixture containing 2.5% of CO₂ with temperatures ranging from 15ºC to 40ºC. The percentage of exhaled O₂ was taken as the same as in ambient air. A quasi-linear variation of approximately 5% was calculated for sound velocity between those two temperatures, corresponding to ca 85 cents increase of resonance frequency variation in an air column.

The following expression can be used for the calculation of the sound speed, as applied by Nederveen [7], for a gas in which small variations in its components occur.

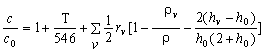(1.1)

(1.2)

where

c = velocity of sound in the gas mixture

c₀ = velocity of sound in typical dry air, at 0ºC

r , r ₀ ,r _v= densities of the mixture, the typical air and the additional gas, respectively

r_v = fractional pressure of additional gas

T = temperature of the mixture, in Centigrade.

h₀ , h_v = number of degrees of freedom of standard air and each additional gas, respectively,

whereby

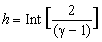(1.4) ;

Int [ ] being the closest integer value of [ ]

2. Materials and methods

The experimental protocol consisted of two different procedures:

(1) measurement of the percentage of CO₂ and O₂ in exhaled air during the playing of long notes, at constant dynamic level and blowing pressure, in the oboe and bassoon. The instruments were regularly played by a musician, starting immediately after a deep breath. The air inside the instrument bore was continuously sampled by an air pump unit (Ametek R-1 Flow Control Device), at a rate of 180 ml/min. The sampled gas flowed through a 1 meter long, 2.5 mm Ø plastic hose inserted into the instrument, with the open extreme located at the medium distance of the air column. The aspirated gas flowed first through a Capnograph (Ametek P-61B Sensor Unit and CD-3A Carbon Dioxide Analyser) and then through the oxygen meter (Ametek N-22 Oxygen Sensor and S-3A/I Analyser). The readings from the devices, and the audio signal were recorded by a multichannel TEAC (RD-200T) PCM DATA recorder, for eventual transfer into a personal computer.

(2) a musical subject blowing through a special mouthpiece device, see Figure 1, which enables the reed to vibrate at a fixed degree of tension, without contact with the player lips, while the exhaled air is kept at fixed pressure. The task consisted of playing a long note after a deep breath, then re-breathing and keeping with long notes (two times in sequence), then taking a 5 seconds break and attacking again (without re-breathing), taking a breath and attacking again and finally doing a circular breathing (see description at discussion section) for a last long note. Blowing pressure was sensed by a calibrated transducer (Gaeltec 7Sb) placed in the mouthpiece device chamber and recorded in the PCM DATA recorder, together with the signals of the gas sensors referred above. A microphone (Sony ECM-959DT) picked up the audio signal, also recorded into a 20 kHz channel.

Figure 1. mouthpiece device which enables the reed to vibrate without contact with the player’s lips. The player blows directly through the air inlet. The adjustable clamp, not shown in detail, incorporates a piece of rubber to press against the reed, thus simulating the effect of the lips. For the bassoon experiment, a similar device was used.

 

 

Experiments were run in a well ventilated room, for which the pre-calibrated analyser showed a reading of 0.03% ambient CO₂. A professional musician (the author) was the sole subject. During the experiment, all output signals, excepted by the blowing pressure readings, were out of the subject’s sight. The visual feedback for the blowing pressure values was necessary as a means of ensuring the subject a fine control for a fixed input pressure.

3. Analysis and results from gas measurements

The recorded signals were transferred from the TEAC recorder into computer files, using the SwellÔ DSP software package installed in a PC computer with an Ariel DSP-16 board. The results were obtained after a simple procedure of calibration, implying that the zero and range of the computer files were adjusted so as to match the corresponding original readings from the CO₂ and O₂ analysers.

Both gases varied continuously during all measurements, and as expected, the CO₂ percentages increased simultaneously to the decrease of O2. The variations for CO₂ percentages ranged between 0% and 8.5%, as previously observed by Fuks [5]. The O₂ contents at the end of long phrases decreased to values of less then 12%, and in some extreme cases reached approximately 11%. These values substantially extend the range as presented by Schmidt & Thews [6], where a minimum of 14% is referred. A typical curve for both gases variations is presented in the upper part of the graph in Figure 2. In that particular case, the subject played the tone E5 in the oboe, using a blowing pressure of 50 cm H₂O. The curves obtained for different tones and blowing pressures all present the same general aspect, with minor differences as a function of playing time and airflow through the reed.

4. CALCULATIONS OF EXPECTED PITCH VARIATIONS

Once the realistic values for the CO₂ and O₂ percentages were obtained during the former measurements, we proceeded with the computation of the expected pitch variations. A spread sheet program was used for implementing expressions (1.1) and (1.2) and plotting the results, shown in the lower part of Figure 2,. A temperature of 25.5ºC was assumed as the equilibrium value for a warmed up instrument, in good agreement with previous measurements in the oboe as 25.6ºC (Meyer, [8]) and in the flute, 26.8ºC (Coltman, [9]). At that temperature, the specific humidity of saturated air is approximately 2.07%.

As can be seen in Figure 2, the expected pitch variations are more pronounced in the early period of blowing after a deep breath. During an initial time interval of approximately 5 seconds, a relevant dip of 15 to 20 cents (approximately one fifth of a semitone) was calculated.

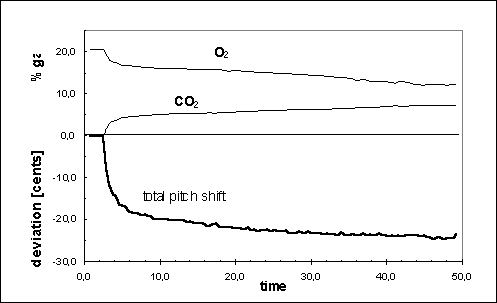
Figure 2 . Measured CO2 and O2 contents (upper part of graph) and computed expected pitch shift(lower part). The zero cent reference corresponds to ambient air. The temperature was assumed as 25.5ºC and the air is saturated with specific humidity of 2.07%.

5. Measurements of variations from performance

Procedure (2) described in section 2 was performed in the oboe and bassoon, with different tones and dynamic levels. The sound files, at a sampling rate of 20kHz, had their fundamental frequency extracted by a signal processing program (FoX-Nyvalla) A typical example of such data is shown in figure 3, the bassoon producing the note B3 with a blowing pressure of 30 cm H₂O, sounding as a mezzoforte tone.

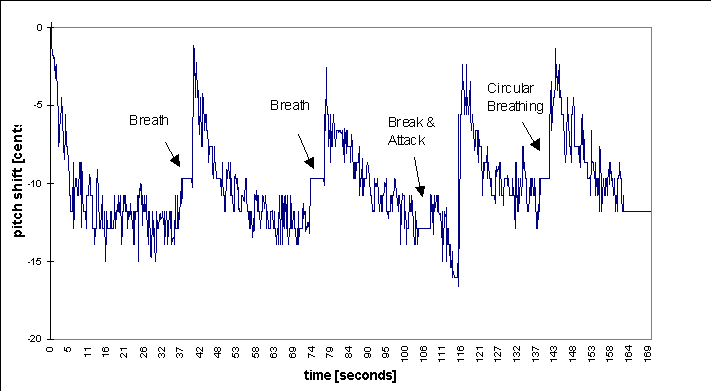
Figure 3. Pitch variations observed during a B3 played in a bassoon(mezzoforte), blowing pressure of 30 cm H₂O. The player used the device depicted in Figure 1, following the procedure described above. The zero reference is defined by the initial pitch at the first attack.

 

 

The curve in Figure 3 is shown in its raw appearance, without any filtering or smoothing. This aspect is due to the program’s algorithm for pitch extraction. A quite similar pattern as shown in Figure 3 was observed in all recordings done with the oboe and bassoon. The pitch invariably decreases from the attack and reaches an almost plateu line. Immediately after a new breath, there is a sudden increase in pitch and a similar pattern is repeated. After a break in the sound, followed by a new attack and without the player re-breathing, the tone re-starts at the same pitch level and keeps decreasing (see Figure 3). When a circular breathing is performed, the air content of the lungs is renewed without the interruption of the sound, and a sudden increase occurs, as in the previous events.

6. Discussion

Our calculations of the effect of gas changes on sound velocity apply only to the feedback instruments, i.e., the instruments in which the source vibrations are dependent on the resonance frequencies of the air column. Thus, pitch effects of the type considered here should not be expected for non-feedback instruments, such as the human voice and the mouth harmonica.

Temperature is generally regarded as a factor of major relevance to the tuning of feedback wind instruments. Thus, warming the instrument, e.g. by playing or by blowing expired air through the bore, is a routine among wind instrumentalists. As explained in a preceding section, a warming from room temperature of 20^oC to 25^oC corresponds to a sharpening of the pitch by about +17 cents. It is interesting that the rarely discussed variations of exhailed air content during playing are comparable to the commonly recognised effects of temperature. However, the variations in pitch due to gas composition are necessarily cyclic, while the temperature effect tends to stabilize with continuous playing.

The formulae used for the air speed calculation (expressions 1.1 and 1.2) apply to small changes in the gas percentages. It is beyond the scope of this article to investigate the error in velocity prediction for the percentages used, but we have reasons for accepting the determined values as the extreme variations in the experiment are of the same order. The pitch variations occurred in our experiment were successfully predicted by our calculations, indicating that the pitch shift due to gas changes is a relevant effect during actual playing.

The mouthpiece device used for the experiment has an internal volume of ca. 40 cm². This fact produces an increase in the anatomical dead space. Probably, it works as a buffer that attenuates at some extent the quick fall in pitch predicted by our calculations.

Presumably, the pitch effects induced by the gas variations are compensated for by the player by means of varying embouchure, blowing pressure and other playing characteristics. The ambient CO₂ and O₂ contents depend on many factors, e.g., the number of people in a room and the air quality. However , if the player is in a room with acceptable levels of ventilation, there is no reason to consider that the effect will reflect in very different results.

In addition to the gas-changes effect one could also argue that on the beginning of a note, if the player has comparatively higher levels of lung volumes, it is much easier to produce higher blowing pressures. Also, blowing pressure affects the sounding pitch in a positive way (Bak and Doemler [2]). We could suggest that the combination of both effects give rise to a strong trend of playing at higher pitch on the beginning of a phrase and a progressive decrease with time (lung capacity), mainly for instruments and tones that require higher pressures. It is a well known fact in human speech production that there is a general trend for the average voice pitch to fall, as the lung volume is reduce and also the subglottal pressure. It would be interesting to investigate if the same phenomenon (f₀ fall) takes place in solo wind playing.

We could propose that the same pitch variation effect will take place in other winds, including brass instruments and the flute. However, due to large differences in the airflow used in each case, there is a need of further experiments for a realistic account of the phenomenon.

Some anecdotal evidence and technical recommendations among professional musicians seems to support the assumption that the predicted gas effect on pitch in wind instruments is realistic. Oboe, clarinet and bassoon players tend to agree that there is a general trend for the pitch of the instrument to drop during long phrases. Particularly for the notes in the lower range of the instruments; it may be very difficult to keep the pitch in tune, even with the use of all possible compensatory efforts. It is generally assumed that this is due to a fatiguing of the muscles involved in playing. While this seems a reasonable assumption, there are also reasons to believe that the gas variations are also important. For example, the use of a quick inhalation or of the so-called circular breathing technique is said to help restoring the desired pitch. Circular breathing is a technique in which the player keeps blowing into the instrument while air exchanges take place through the nose. To accomplish it, an amount of air is saved in the mouth cavity, isolated from the remaining airways by pressing the soft palate against the back of the tongue. Also, many professional players recommend exhalation of a small volume of air just after the inhalation and before starting a tone (e.g. Robinson [10]). This procedure is usually claimed to improve production of soft attacks, facilitate intonation control and create a more relaxed respiratory feeling.

7. Conclusions

During playing wind instruments such as the oboe and the clarinet, the content of CO₂ in the expired air varies between the ambient level and up to 8.5% in extremely long phrases, while for the O₂ it may vary from the ambient 21% down to 12%, or even less. The increase in CO₂ tends to decrease the pitch and the fall in O₂ tends to increase pitch. Even then, due to differences in gas properties and the rate with which the gases vary, the total effect is that of pitch decrease. This effect may account for a fall in fundamental frequency of the tones by more than 20 cents. Although we could presume that the pitch effects induced by gas variation are compensated for by the player by means of varying embouchure, blowing pressure and other playing characteristics, this effect still seems a relevant factor in wind instrument playing.

8. Acknowledgements

I am very grateful to Prof. Johan Sundberg, for the stimulating and fruitful discussions on this theme and the assistance in preparing this manuscript. I highly appreciate the biomedical information provided by Doctors Miriam Katz-Salomon and Michael Runold from the Karolinska Institute as well as the opportunity of using the equipment from the same Institute. This study was supported by the Brazilian Ministry of Education (CAPES Foundation).

9. References

[1] Porter M ,’The Embouchure’, London: Boosey and Hawkes. (1973)

[2] Bak N and Doemler, ‘The Relation between Blowing Pressure and Blowing Frequency

in Clarinet Playing’, Acustica vol. 63, p.238-241 (1987)

[3] Fuks L & Sundberg J ,’Blowing pressures in reed woodwind instruments’, KTH TMH-

QPSR 3/1996, Stockholm, 41-56 (1996)

[4] Zenz Carl ,’Occupational Medicine’, 2^ND edition. Year Book Med Publ Inc (1988)

[5] Fuks L ,’Prediction of pitch effects from measured CO₂ content variations in wind

instrument playing’, KTH TMH-QPSR 4/1996, Stockholm, 37-43(1996)

[6] Schmidt RF & Thews G, eds ,’Human Physiology’ New York: Springer-Verlag. (1983)

[7] Nederveen C ,’Acoustical Aspects of Woodwind Instruments', Amsterdam: Frits Knuf;

15-20 (1969)

[8] Meyer J ,’Über die Messung der Frequenzscalen von Holzblasinstrumenten’. Das

Muzikinstrument 10: 614-616 (1961)

[9] Coltman JW, ‘Resonance and sounding frequencies of the flute’, J Acoust Soc America, 40/1: 99-107, (1966)

[10] Robinson J ,’Oboists, exhale before playing’, Double Reed. International Double Reed

Society, re-published in Vol 19/3, (1996)