
This investigation was focused on reed woodwind instruments and on voice, the latter with respect to a particular effect of vocal-ventricular phonation. Limiting the instrumental study to four types of instruments was due to practical and methodological considerations. A practical consideration was the huge amount of experiments and data processing required to achieve some degree of depth in the analysis of a given instrument. A methodological consideration was the vast differences in the principles that regulate the sound generation in different types of wind instruments, e.g., the above-mentioned mechanical reed, free reed (harmonica, accordion), air-reed (flutes, recorders, etc.) and lip-reed (brass) instruments. These differences lead to separate playing techniques, necessitating the use of different theoretical approaches for each instrument type. Nevertheless, most of the studies and procedures in this work might be useful in future investigations of other types of wind instruments.
The idea to include the human voice in this work originated from the observation of some apparent similarities between the mechanical system of reed instruments and certain properties of the specific phonation mode studied. These similarities will be explained in greater detail below.
5a Reed woodwinds

Figure 2. Bassoon plant. Despite standard and accurate manufacturing procedures adopted by the maker, the different parts of an instrument must be mutually adjusted, so that equivalent parts are not usually interchangeable. The instruments shown incorporate the German Heckel system (Blei & Baumann, 1987).
Mechanical reed woodwinds constitute an important group of wind instruments, which in western classical tradition includes oboes, clarinets, saxophones and bassoons. In these instruments, the function of the reed is to modulate the airstream that enters the air-column of the instrument, exciting and maintaining the vibrations in the bore.
Each of the instruments are available in two or more "voices", according to pitch range: soprano oboe, oboe d’amore, English horn; soprano clarinet (in Bb and in A), Eb clarinet, basset horn, bass clarinet; soprano, alto, tenor, bass saxophones; bassoon and contra-bassoon. There are still more variants. Usually, different voices of an instrument use almost identical fingerings. There are, however, instruments designed with different key systems and tonehole configurations, particularly for the oboe and the bassoon, which may also differ in sound quality and in playability, see Figure 2.
Among the four instrument types, we selected the four most commonly used, shown in Figures 3-6. The reeds for the alto soprano and the clarinet are very similar, the first being larger in all dimensions, see Figure 7. The reeds for all four instruments are generally made of a species of bamboo, Arundo donax. Other, mainly synthetic, materials are also available. However, the bamboo is by large the most common among professional classical users. Since oboe and bassoon reeds are autonomous pieces, directly attached to the instrument bore, they will be referred to as reeds or mouthpieces. Figures 3 to 7 from Nederveen (1969).
|
|
Figure 4. Soprano clarinet |
|
Figure 5. Bassoon |
Figure 6. Alto-saxophone |

Figure 7. A clarinet/saxophone mouthpiece. The reed is rigidly clamped by the ligature in one extreme. The blade is free to oscillate along the curved profile of the mouthpiece. The airflow slit is the varying opening area between the reed margins and the mouthpiece lay. Embouchure sets a different contact region between the parts, moved towards the tip, characterised by a less rigid and more damped attachment (see Figures 10a and b).
Reed woodwind instruments produce tones at pitches which are dependent on many factors: the length of the acoustical air-column inside the instrument, the shape of the instrument bore, the sound speed of the air inside the instrument, the natural vibrating frequencies of the reeds and, to some extent, the blowing pressure. Also, the so-called embouchure is highly influential. The term embouchure is somewhat vague (Porter, 1967; 1973). In this thesis it is assumed to be the constellation of forces and positions in the lips, mouth region and face that act on the instrument. A more detailed account for the effects of the embouchure is given in the end of this section.
The length of the air-column is mainly determined by the fingering applied to the instrument mechanism, which permits a large combination of open and closed tone-holes. This length can be modified by the longitudinal position of the mouthpiece, which allows fine tuning adjustment of the instrument with respect to an intonation reference. The tuning is also affected by the acoustical length of the reed, which depends on the physical reed length and shape.
The shape of the instruments' bore may be roughly approximated to a cylinder in the clarinet and to a truncated cone in the oboe, saxophone and bassoon. In simplified terms, the sound waves travelling in a cylindrical tube closed at one end, such as in an idealised clarinet, may be considered as quasi plane. The frequencies of the resonance modes approach a harmonic series containing the odd multiples of the lowest resonance. For a conical tube, the waves produced are quasi spherical, thus a series comprising all multiples of the lowest resonance frequency. In reality, those resonance modes present inharmonicities, i.e. they are not exact integer multiples of the lowest resonance (Bouasse, 1929; Benade, 1968; Gilbert, 1991).
The speed of sound propagation in the instrument is of great importance. The reason is that the reed oscillations are controlled by a vibratory regime in the bore; acoustic pulses, created by the reed’s modulation of the airstream from the mouth, travel along the bore, are reflected at the effective end point of the air-column and return back to the reed chamber. The travel time depends on the length of the bore and of the sound speed. This speed depends on the temperature, humidity and the composition of the gas inside the instrument, which thus affect the fundamental frequency of the tones, see Figure 8. The magnitude of these effects is investigated in detail in Papers IIIa and IIIb.

Figure 8. Players must adapt themselves to perform under various conditions. At low temperatures, the normal fundamental pitch of a wind instrument should decrease considerably, for example, almost a semitone at -10°C. However, as the instruments are warmed up, this deviation should be greatly reduced. Photo by Blei & Baumann (1987).
The reeds generally oscillate at frequencies that are far below their inherent frequencies, i.e. frequencies at which they would vibrate should they not be coupled to an air-column (Helmholtz, 1863; Bouasse, 1929; Benade, 1976). For instance, when the player increases the load forces in the embouchure, thus increasing the reed stiffness, the inherent frequency of the reed is raised. This increases to some extent the sounding pitch. Players continuously use this principle for fine adjustments of intonation. Also, in clarinets and saxophones the single reed is bent against the curved lay of the mouthpiece, see Figure 7. This implies an increase in load force and a shortening in the vibrating length of the reed, which raises the fundamental frequency to some degree. This affects the equivalent length of the instrument (Gilbert, 1991, and see below). "Squeak" sounds, high-pitched sounds that accidentally occur mainly in the clarinet, are explained as a consequence of an insufficient coupling of the reed to the air-column modes. In this case, the reed vibrates closer to its natural frequency, occasionally reinforced and assisted by a resonant peak of the mouth cavity and/or the instrument bore. "Squeak" sounds are sometimes used in contemporary music, often controlled by the direct contact of the teeth on the reed (Rehfeldt, 1994) and/or by skilful shaping of the vocal tract.
Apart from the embouchure’s effect on reed stiffness, it probably also adds to its effective mass. The surface of the lips that touches the reed should form a layer of tissue that participates in the oscillations. This should reduce the natural frequency of the reed, implying that the fundamental frequency should decrease somewhat. This seems to be a neglected factor in previous investigations. In a pilot experiment, we applied a thin film of plastic gum of 0.1 g on both sides of a bagpipe reed, connected to its air-column (a conical chanter), blowing at constant pressure. This caused the fundamental frequency to decrease by more than 15 cents. The bagpipe reed is located inside a cap, isolating it from the lips. This result suggests that the mass added to the reed by the lips is significant.
Blowing pressure is intimately related to airflow in reed woodwinds. Analysis of the static airflow, void of oscillation, across a reed-like device has shown characteristic relations between the two, see Figure 9 (from Fletcher and Rossing, 1998, after Wijnands & Hischberg, 1995 and Worman, 1971). They reveal that, for a given embouchure, airflow varies with pressure according to a bell shaped curve. Acoustical and aerodynamical considerations show that self sustained oscillations will only be possible on the descending side of the curve and that these oscillations can be started at a threshold pressure, i.e., approximately one third of the pressure that completely closes the reed. The pressure-flow curves are different for double reeds, such as oboes and bassoons, although similar principles apply. The differences consist of the fact that in curve III there is hysteresis, implying that an increase of the upstream pressure to a maximum will suddenly interrupt the flow, while a different behaviour occurs when going the reverse direction.
In practical terms, the static flow curves show that for a fixed embouchure the airflow and the sound power radiated by the instrument should decrease with increasing blowing pressure. Papers I and II investigate the pressure and airflow in naturally played instruments.
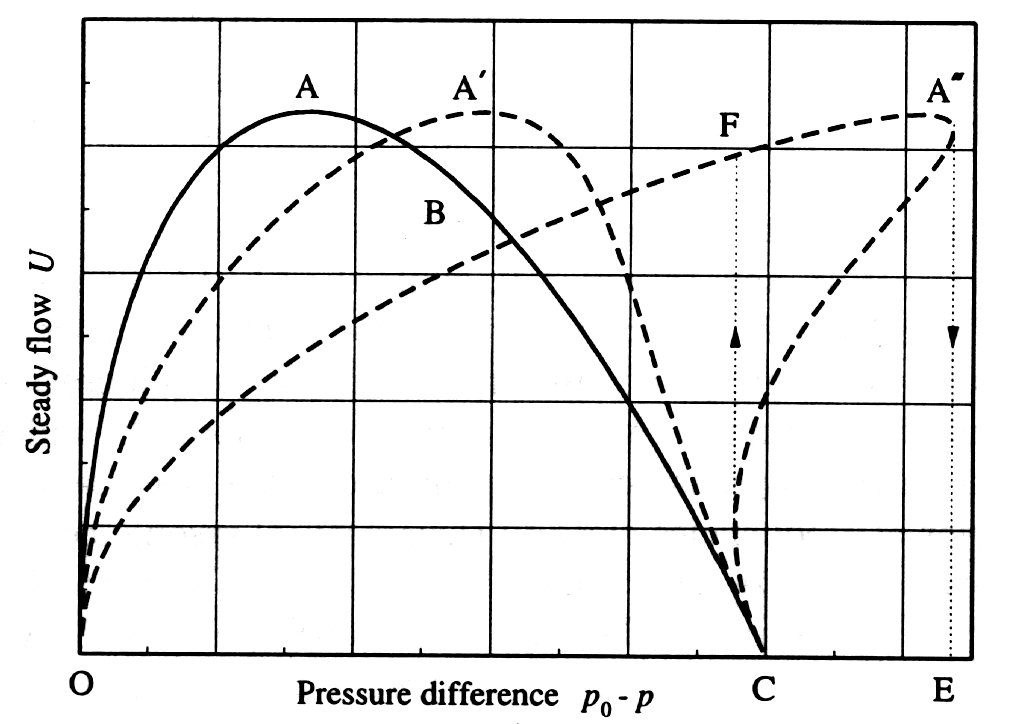
Figure 9. Pressure-flow curves through a static reed, i.e. without oscillations. With a blowing pressure of p0 and an internal pressure of p, flow increases from O until it reaches a maximum at A and decreases until the closure point C. For the two curves on the left, the instruments can only operate in the descending part of the bell-shaped curves. Filled line represents the typical case of the clarinet (Worman, 1971). Double reeds may behave according to one of the three curves, because of internal flow resistance. From Fletcher & Rossing (1998), after Wijnads & Hirschberg (1995).
Another important phenomenon occurring in instruments is overblowing. In reed woodwinds it refers to the situation that the reed vibration frequency is controlled by a higher oscillation mode of the air column. Overblowing is caused by a particular combination of embouchure and input parameters, sometimes assisted by a special fingering. As mentioned above, conical bores have modes at approximately integer multiples of the frequency of the lowest mode. Thus, overblowing will make the reed oscillate at higher resonance modes in the air-column. Similarly, in conical bores overblowing establish reed vibrations at frequencies which are about 3, 5, 7 etc. times the lowest mode. The overblowing phenomenon delimits the different registers of the instrument. Generally, the reed instruments use no more than three registers but under special circumstances, additional registers may occur.
The oboe, Figure 3, has a length of approximately 644 mm, including the reed, and its usual range is Bb3-G6. The first register is limited by the note C5. The second runs up to C6, and has fingerings very similar to those in the first register. The fingerings in the third register are less systematic for reasons of tuning. The double reed, consisting of two opposed, curved blades tied to a metal staple, is shown in more detail in the same figure. Of paramount importance is the design and finishing of the reed scrape, as it affects the playing properties of the reed. Usually, oboe and bassoon players make and adjust their reeds according to the instruments, the material properties, and to personal preference.
The Bb clarinet, Figure 4, has a bore measuring about 664mm and is approximately cylindrical. The instrument typically ranges between D3 and B6. Ab4 limits the first register. Because its air-column modes are odd multiples of the fundamental mode, the second register gives three times the fundamental frequency, in musical terms a duodecim or a twelfth. This register is available from A4, which uses the fingering of D3 plus the register hole. This register continues up to Bb5 (position corresponding to Eb4). From this point on, the third register follows until B6, with non-sequential fingerings as in the former registers. Only Boehm system clarinets, the most widespread, were used in our experiments.
The bassoon, Figure 5, like the oboe, consists of a conical tube with a double reed, measuring approximately 2560 mm and ranges typically between Bb1 and D5. The first register spans from Bb1 to F3. This is considerably larger than the other instruments. From F#3 up to D4, the fingerings are similar to the previous octave, with the assistance of some keys. Then, the last octave comprises fingerings that vary greatly. In our experiments, all bassoons belonged to the German (Heckel) system, see Figures 2 and 5.
The alto saxophone, Figure 6, consists of a quasi-conical bore of 1062 mm and a typical playing range between Db3 to A5. The first register reaches E4, the second register covers the range F4 to A5, and uses fingerings similar to those used in the first register. For saxophones, mostly one single system is used.
Embouchure
From the above, it is evident that the embouchure serves many different purposes, all controlled by the player. Figure 10b presents a tentative schematic model for how the mechanical parameters of the embouchure are distributed along the reed. The lips are pressed against the reed with a load force distributed on the surface (dFm). The tissue has a stiffness (dKm) and a mass distribution (dMm), and also attenuates the oscillations with a damping coefficient (dRm).
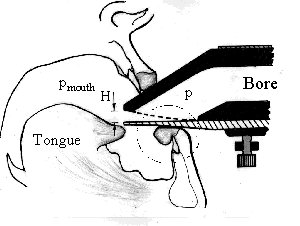

Figures 10a and 10b. Simplified representation of the embouchure (a) and a detail with its mechanical parameters (b) on a single reed instrument. For double reeds, obviously both blades will be in contact with the lips and the factor of the reed bending along the mouthpiece lay is not present.
The purposes of the embouchure are as follows:
By modulating the embouchure, usually accompanied by a modulation of the blowing pressure, it is possible to produce a gamut of sound effects and gestures, including the so-called "lip vibrato", briefly discussed in Paper II.
It must be stressed that the above descriptions refer to embouchure in reed woodwinds. In the case of brass instruments, where the lips serve as the main oscillator, such function must be added and the purposes represented by items b, c and d do not apply. Also, the embouchure in air-reed instruments, such as flute and recorder, require a different description.
In defining the embouchure, it is possible also to include factors that determine the intraoral configuration, such as the position of the jaw and tongue. The shape of the mouth cavity is frequently assumed to be relevant to the sound production (Benade, 1986; Thomas et al., 1988; Backus, 1963b).
5b Human voice: Ventricular fold phonation
Human voice might be regarded as a special case of wind instrument. The source oscillations are mainly defined by the inertial and viscoelastic properties of the vocal folds and the aerodynamic parameters of the airflow passing them, while the feedback from the air column upon the vocal fold oscillator is generally of minor significance. Yet, a particular type of voicing seems interesting from the point of view of wind instrument acoustics, viz. the one produced by some Tibetan monks and ethnic groups of Central Asia. This type of voicing is characterised by a very low fundamental frequency and a loud tone, rich in harmonics as compared to corresponding tones produced by Western bass singers (Smith et al., 1967; Barnett, 1977; Dmitriev et al., 1983; Campbell & Greated, 1987; Zemp, 1996). The underlying mechanism has not been clearly explained. This raises the question whether structures other than the vocal folds could play the role of an oscillator.
It seemed reasonable to assume that the ventricular (or false) vocal folds vibrate in such voice production. The geometrical configuration of these structures differs considerably from that of the vocal folds. As can be seen in Figure 1, Paper VI, the ventricular folds have a downward angulation. Thus, they can serve as a closing valve, preventing exhalation at high intrathoracic pressures, such as during the initiation of coughing. The vocal folds have the opposite angulation, so that they can serve the purpose of closing the airways during inhalation, such as in hiccup. According to Helmholtz (1863), the valve systems represented by the reeds in musical instruments may be classified into inwards striking "tongues", or valves (e.g. reed woodwinds), and outwards striking valves (brass instruments and voice). An interesting possibility studied in Paper VI was if they then function as an inward closing valve, i.e., similar to a woodwind reed.
©1998 by Leonardo Fuks